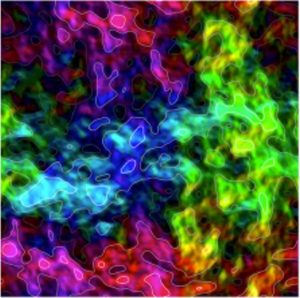
Active matter is composed of particles able to transform available energy into work, enabling them to generate their own motion and to interact each other. Depending on the symmetries of the system, external or inter-particle interactions, and the level of fluctuations, it is possible to form long-distance organized phases.
Active particles can form structures that differ from those formed at equilibrium by passive particles. The present study, published in Physical Review Letters, focuses on two-dimensional systems, and shows the formation of active matter crystals that exhibit extreme large-scale spontaneous deformation, without reaching fusion. This example illustrates the limitation to thermodynamic equilibrium conditions of the predictions of Kosterlitz-Thouless-type theories.
In statistical physics, the phase diagram depends on the dimensionality of the system. Two-dimensional crystals have long fascinated theorists, because it was possible to establish exact solutions, and for their specific properties. There are many experimental examples, such as electrons on the surface of helium, the rough transition of the surface of single crystals, or a set of colloidal particles confined to an interface. Highly sensitive to fluctuations, ordered states are rather fragile and exhibit only quasi-long-range structural order.
Much of the theorists’ fascination with these 2D systems lies in the tour de force represented by a series of papers published in the 1970s by Kosterlitz, Thouless, Halperin, Nelson and Young. Their “KTHNY” theory describes the phase diagram of the system. It shows mainly that 2D crystals possess a long-range order for the orientation of bonds between neighboring particles, and that their melting can occur in two stages. When continuous, these two transitions are of the Kosterlitz-Thouless type, i.e. they exhibit singularities at all orders (infinite order). The first transition marks the loss of positional order due to the loss of bonding between defect pairs in the form of dislocations, leaving a phase with only one quasi-long-range bonding order. KTHNY theory also predicts that the decay exponent h, characterizing the decay with distance of the long-range positional order in the crystal, increases continuously with temperature, but cannot be greater than 1/3.
The KTHNY theory is not supposed to apply out of thermal equilibrium, although some recent papers claim it does in the context of “active” particles, i.e. a system composed of particles moving along an intrinsic physical axis, and which therefore cannot be instantaneously disordered.
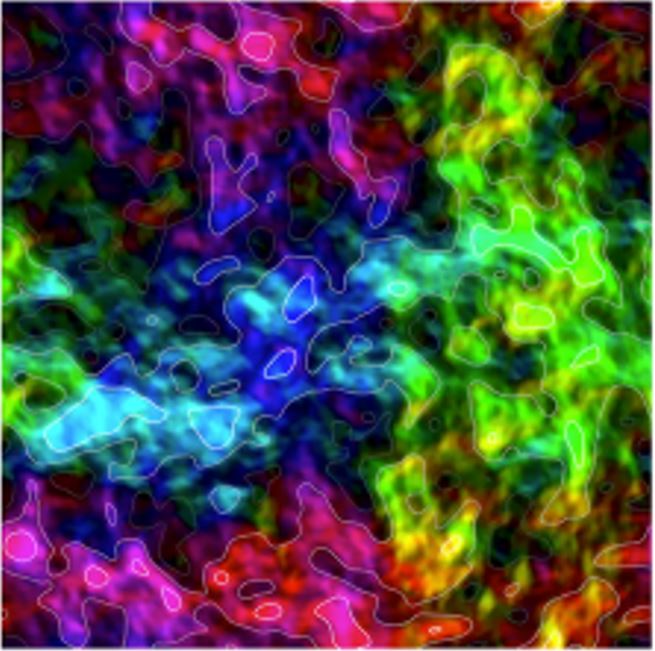
In a recent paper published in Physical Review Letters [1] and reprinted in Physics Magazine, Hugues Chaté and colleagues from Suzhou, China, show that 2D crystals of active particles can in fact undergo extremely large deformations before reaching the molten state, with an exponent h that can take on virtually unlimited values. These large deformations are made possible by the persistence of the intrinsic axis of the active particles. Similarly, a crystal of passive particles (i.e. with no preferred axis of displacement), but subjected to “persistent” (non-Brownian) fluctuations, is also rendered extremely deformable, while remaining free of free defects.
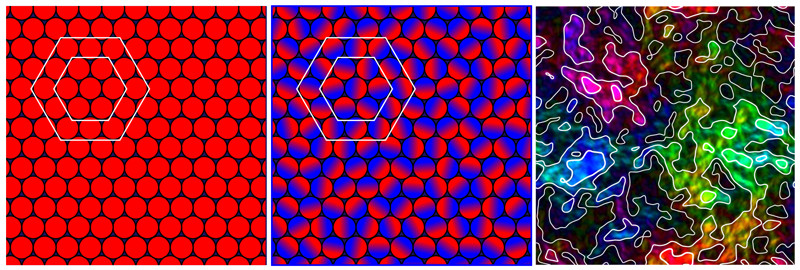
Left: at equilibrium, a two-dimensional system of particles stabilizes in an ordered structure with long-range order that looks like a crystal lattice. Center: active 2d systems stabilize in a solid-like ordered phase, but with extremely large fluctuations around the perfect crystal lattice. Simulations (right) show that the amplitude of these fluctuations can exceed several lattice parameters (color is a function of displacement orientation and intensity is a function of amplitude).
The studied example provides evidence that KTHNY theory cannot be extended to non-equilibrium situations, at least on a quantitative level. These results can be applied to macroscopic mechanical meta-materials that are also made up of active elements and can resist large deformations without affecting the integrity of the material.
[1] Extreme spontaneous deformations of active crystals
Xia-qing Shi, Fu Cheng, and Hugues Chaté Phys. Rev. Lett. 131 (2023) 108301.
See also “Viewpoint APS Physics ” : “Active particles push the boundaries of two-dimensional solid” by Mathias Casiulis.
Contact: Hugues Chaté, SPEC/SPHYNX
Collaboration :
- Université Paris-Saclay, SPEC/SPHYNX, UMR 3680 CEA-CNRS, CEA-Saclay, 91191 Gif-sur-Yvette, France
- Center for Soft Condensed Matter Physics and Interdisciplinary Research, Soochow University, Suzhou 215006, China
- Computational Science Research Center, Beijing 100094, China.