Logo CEA
Recherche
Observation of magnetic fragmentation in spin ice

Observation of magnetic fragmentation in spin ice

Fractionalized excitations that emerge from a many-body system have revealed rich physics and concepts, from composite fermions in two-dimensional electron systems, revealed through the fractional quantum Hall effect, to spinons in antiferromagnetic chains and, more recently, fractionalization of Dirac electrons in graphene and magnetic monopoles in spin ice. Even more surprising is the fragmentation of the degrees of freedom themselves, leading to coexisting and a priori independent ground states.

This puzzling phenomenon was recently put forward in the context of spin ice, in which the magnetic moment field can fragment, resulting in a dual ground state consisting of a fluctuating spin liquid, a so-called Coulomb phase, on top of a magnetic monopole crystal. In a Nature Physics publication it is reported that, by means of neutron scattering measurements, such fragmentation occurs in the spin ice candidate Nd2Zr2O7. A spectacular coexistence of an antiferromagnetic order induced by the monopole crystallization and a fluctuating state with ferromagnetic correlations is observed. Experimentally, this fragmentation manifests itself through the superposition of magnetic Bragg peaks, characteristic of the ordered phase, and a pinch point pattern, characteristic of the Coulomb phase. These results highlight the relevance of the fragmentation concept to describe the physics of systems that are simultaneously ordered and fluctuating.

Spin ice is an unusual magnetic material in which the combination of strong local Ising anisotropy and ferromagnetic interactions imposes a local constraint to the magnetic moments, the “ice-rule” (see figure 1a). The magnetic state thus remains disordered but with specific local correlations. It belongs to the class of Coulomb phases, an original state of matter where the ground state is disordered, and the local constraint can be interpreted as the flux conservation law of electromagnetism.

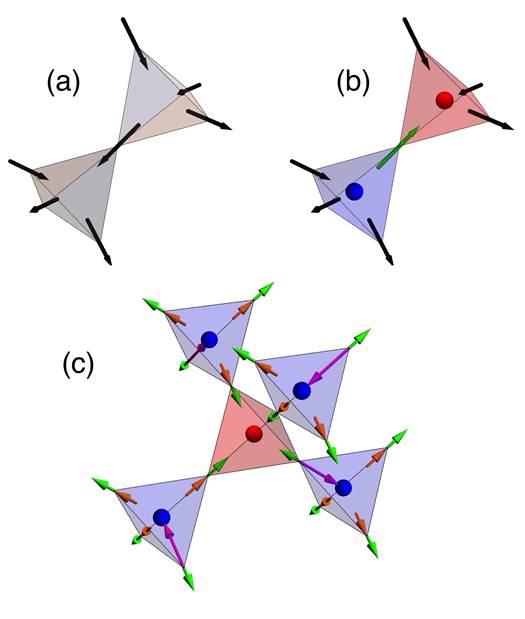
Figure 1: (a) The spin-ice “two-in two-out” configuration of the spins (arrows). (b) A spin flip (green arrow) creates 2 magnetic monopoles in the centre of the tetrahedra (read and blue dots). (c) The fragmented moments superimposed on the lattice. They are composed of “all-in all-out” (green arrows) moments from the crystallized monopoles and of the fluctuating (orange and purple arrows) spins from the (spin-liquid) Coulomb phase.

Classical excitations in spin ice, called magnetic monopoles, are defects that locally violate the ice rule by reversing the orientation of a moment (see figure 1b), and thus induce a magnetic charge in the electromagnetic field. When the density of monopoles is large enough, an outstanding effect has been predicted by Brooks-Bartlett et al [1]: the emergent charged field can fractionalize into two parts, through the so-called “magnetic fragmentation” mechanism.

We have observed such “magnetic fragmentation” in a recently discovered material, the spin ice candidate Nd2Zr2O7, by means of neutron scattering measurements. This fragmentation (see figure 1c) manifests itself as a striking coexistence between an antiferromagnetic ordered state and a Coulomb phase with ferromagnetic like correlations. Experimentally, it manifests as the superposition of magnetic Bragg peaks, characteristic of the ordered phase, and a pinch point pattern, characteristic of the Coulomb phase (see figure 2). We further show that these two fragmented parts of the magnetic moment field behave independently.

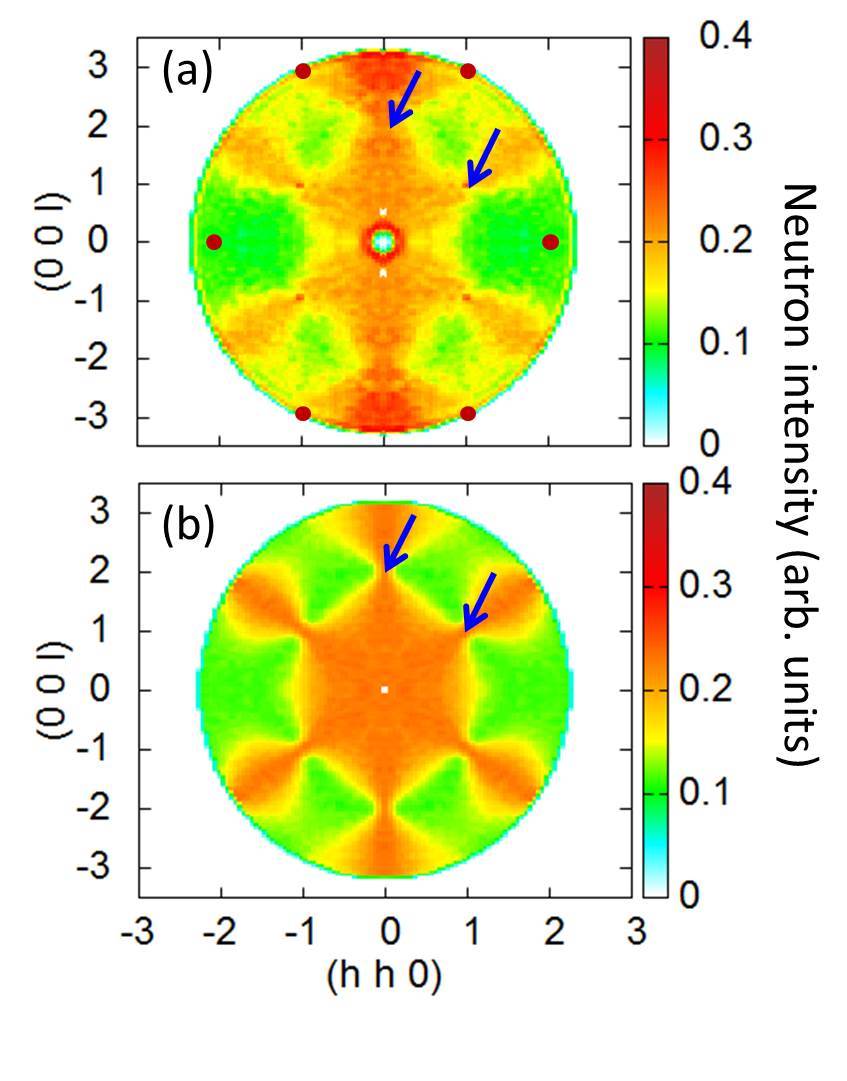
Figure 2: Iso-energy inelastic intensity (E = 50 μeV) showing the pinch points (blue arrows) that are characteristic of the fluctuating fragment. Top : experimental, bottom : numerical simulation.

Behind these observations, our experiments demonstrate that the electromagnetic field is “real” in these magnetic materials and highlight the relevance of quantum effects. They open a new route in the study of frustrated magnets.

The work could only be achieved through a series of neutron scattering techniques: neutron diffraction on the D23-CEA-CRG diffractometer to observe the ordered fragment, polarized diffuse scattering and inelastic scattering on the D7 and IN5 spectrometers to observe the fluctuating part and the inelastic process. It is a collaboration between the LLB (CEA-CNRS-Univ. Paris-Saclay), CNRS Grenoble, Warwick University, CEA Grenoble and the ILL.

Reference:

[1] Magnetic-moment fragmentation and monopole crystallization.
M. E. Brooks-Bartlett, S. T. Banks, L. D. C. Jaubert, A. Harman-Clarke, and P. C. W. Holdsworth
Phys. Rev. X 4, 011007 (2014).

[2] Observation of magnetic fragmentation in spin ice,
S. Petit, E. Lhotel, B. Canals, M. Ciomaga Hatnean, J. Ollivier, H. Mutka, E. Ressouche, A. R.Wildes, M. R. Lees and G. Balakrishnan,
Nature Physics (2016), published on line.

Collaboration:

Luc BARBIER