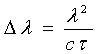•  Interaction laser-matière ›
Interaction laser-matière ›  Interaction laser-matière en champ fort
Interaction laser-matière en champ fort

High-order harmonic generation in gases (HHG) is now recognized as a very interesting source in the XUV range, typically from 100 to 10 nm but even down to the water window at 3 nm. HHG is relatively easy to produce under conditions of ultra-short pulses (t~100 fs) of “intermediate” intensity (I~1015 Wcm-2), with either the LUCA and UHI lasers in Saclay. Harmonic light gets most of its unique characteristics from the fact that HHG is a coherent process tightly driven by the laser field. In addition to the ultra-short pulse duration and high repetition rate, the temporal and spatial coherence, regular wave front, or mutual coherence of two harmonic sources originate in the corresponding properties of the driving laser.
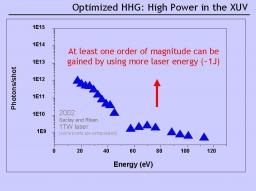
1. Optimized harmonics: up to microjoules !
A large part of our group activity is related to the optimization of the high harmonics flux. We have recently shown that using a "loose focusing" geometry the harmonic efficiency can be strongly enhanced. Using a 5 m focal length, up to 25 mJ (compared to 2 m, up to 5 mJ) in xenon, we have obtained a gain of a factor 6 in the harmonic conversion efficiency and a factor 40 in the number of photons produced. The harmonic energy per pulse is about 2µjoules at 53 nm, pulse duration is about 10 to 20 femtosecond, beam divergence is about a few mrad. We propose for the next few years to increase significantly the photon flux by at least one order of magnitude using high energy lasers (up to 1J, 10Hz)) and to develop extended procedures for active control of the spatial, temporal and spectral properties of the harmonic emission (pulse shaping).
2. Coherence properties
HHG gets its coherence properties from the laser driving field. Under conditions close to phase matching, the harmonic phase is, at a first approximation, determined everywhere in space and time by the phase and intensity of the laser. This is the basis for the properties of intrinsic, spatial and temporal coherence of the harmonic field. However, for a greater understanding, one should consider that the coherence of the laser field itself (the non linear polarization) is affected by the onset of any time- and space-dependent process in the generating medium, such as ionization and subsequent electronic dispersion. Moreover, one should account for the intensity-dependent dipole phase Fq in the harmonic field, which can also degrade both the spatial and temporal coherence of the harmonic emission. As a result, the coherence properties are critically dependent on the generation parameters [Salières 1998,1999a, Le Déroff 2000a].
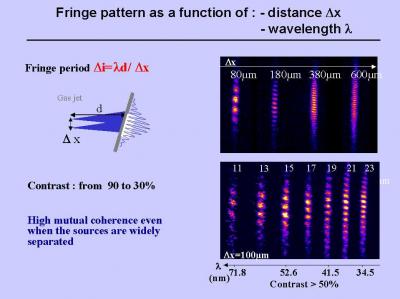
Even in the case of a reduced intrinsic coherence of a single harmonic source, the key feature of a laser-driven process enables two mutually coherent harmonic sources to be produced (see figures below). This remarkable property is highly specific to the HHG. We have successfully used it to perform interferometric applications of the harmonic light .
Spatial coherence of the harmonic emission
We have measured the complex degree of spatial coherence for harmonics 13th and 15th generated in a Xe gas jet, using a Fresnel’s mirrors interferometer [Le Déroff 2000b]. With this arrangement, we can build a 2D-map of the coherence throughout the transverse cross-section of the harmonic beam, for a given separation d between the interfering rays, at distance L»1 m from the source. For the optimal set of phase-matching parameters, a high degree g12 g12(modulus), g ³0.5, is found for separation d up to the full diameter of the harmonic beam (~3 mm): it means that the coherent flux is almost equal to the total flux. Because it reflects the intrinsic coherence of the harmonic source, the g12 degree is much larger than that obtained in similar conditions from a purely incoherent source ( g12 » 0.02 for d=3 mm), the latter being that of most XUV sources. Figure 1 shows the variation of the g12 degree as a function of the jet-to-focus relative position, for distance d=2 mm between the interfering rays. The decrease of g12 when moving the focus from outside (|z|>2 cm) to inside the jet is due to the high laser intensity and subsequent ionization in the generating medium, resulting in large space- and time-dependent factors in the harmonic field phase and reduced coherence.
Mutual coherence: Generation of XUV phase-locked sources
Two mutually coherent harmonic sources can be produced from two mutually coherent laser pulses, either separated in space or in time. The former scheme corresponds to Young’s two-slit and has been demonstrated for high harmonics in the Lund group [Zerne 1997]. We have developped this thechnique (J.-F. Hergott et al. Laser and Particle Beams 19 (1) 35 (2001)) and applied it for inteferometry measurement (D. Descamps et al., Optics Letters 25 (2) 135 (2000)).
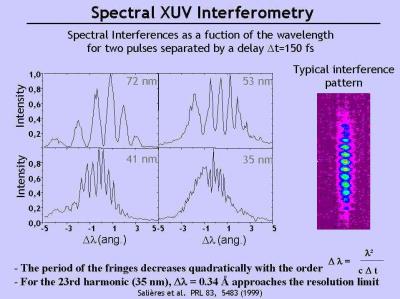
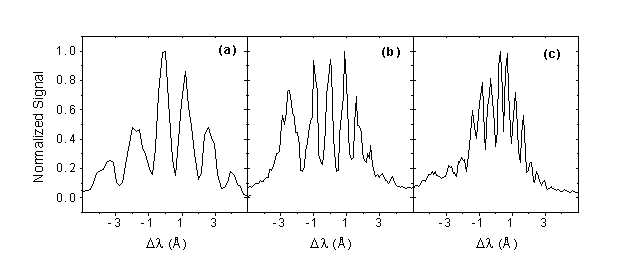
This scheme is known as frequency-domain interferometry and currently used in the visible [Colombeau 1990]. We have demonstrated that the technique can be extended to the XUV using harmonics (P. Salières el al. Phys. Rev. Lett. 83, 5483 (1999). Two harmonic pulses separated by a delay t are produced at the same place in the medium by two delayed but spatially superimposed and otherwise identical laser pulses. After diffraction on a spectrally dispersive optics (grating), the two pulses overlap in time and interfere in the frequency domain. Figure 2 shows the interference pattern measured in the spectral plane for 11th , 15th and 19th harmonic pulses delayed by t=120 fs. The large contrast indicates that, when ionization by the first laser pulse is not too high, the two harmonic sources are mutually coherent. Conversely, the study of the contrast as a function of the generation parameters gives information on the dynamics of ionization in the medium.
We have used this scheme to perform spatial interferometry for studying the expansion of laser created plasmas (D. Descamps et al. Optics Letters 25 (2) 135 (2000) (P. Salières el al. Phys. Rev. Lett. 83, 5483 (1999)).